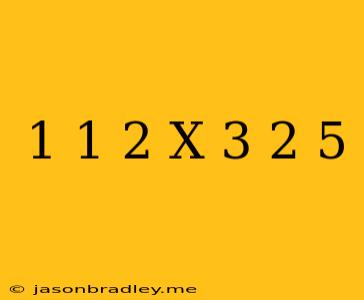Multiplying Mixed Numbers: 1 1/2 x 3 2/5
This article will guide you through the process of multiplying mixed numbers, using the example of 1 1/2 x 3 2/5.
Understanding Mixed Numbers
Mixed numbers combine a whole number and a fraction. To multiply mixed numbers, we first need to convert them into improper fractions.
Converting Mixed Numbers to Improper Fractions
1. Multiply the whole number by the denominator of the fraction:
- For 1 1/2, 1 x 2 = 2
2. Add the numerator of the fraction to the result:
- 2 + 1 = 3
3. Keep the same denominator:
- The improper fraction for 1 1/2 is 3/2.
4. Repeat the process for the other mixed number:
- For 3 2/5, 3 x 5 = 15
- 15 + 2 = 17
- The improper fraction for 3 2/5 is 17/5.
Multiplying Improper Fractions
Now that we have our improper fractions, we can multiply them like any other fraction:
1. Multiply the numerators:
- 3 x 17 = 51
2. Multiply the denominators:
- 2 x 5 = 10
3. Simplify the resulting fraction:
- 51/10 is an improper fraction. We can convert it back to a mixed number: 51 divided by 10 equals 5 with a remainder of 1. Therefore, the simplified answer is 5 1/10.
Conclusion
Multiplying mixed numbers involves converting them to improper fractions, multiplying the fractions, and then simplifying the result. Following these steps, you can successfully multiply any mixed numbers.