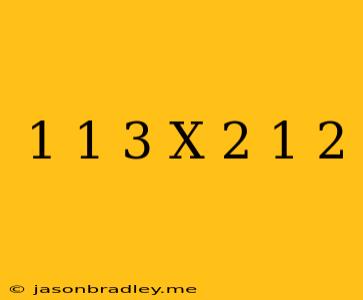Multiplying Mixed Numbers: 1 1/3 x 2 1/2
Multiplying mixed numbers might seem daunting, but it's actually a straightforward process. Let's break down how to solve 1 1/3 x 2 1/2.
Step 1: Convert Mixed Numbers to Improper Fractions
- 1 1/3: Multiply the whole number (1) by the denominator (3) and add the numerator (1). This gives you 4. Keep the same denominator (3). So, 1 1/3 becomes 4/3.
- 2 1/2: Multiply the whole number (2) by the denominator (2) and add the numerator (1). This gives you 5. Keep the same denominator (2). So, 2 1/2 becomes 5/2.
Step 2: Multiply the Fractions
Now that we have improper fractions, we simply multiply the numerators and the denominators:
(4/3) x (5/2) = (4 x 5) / (3 x 2) = 20/6
Step 3: Simplify the Answer
The fraction 20/6 can be simplified. Both 20 and 6 are divisible by 2:
20/6 = 10/3
Step 4: Convert Back to a Mixed Number (Optional)
The answer in its simplest form is 10/3. However, you can also convert it back to a mixed number:
- Divide the numerator (10) by the denominator (3): 10 ÷ 3 = 3 with a remainder of 1.
- The quotient (3) becomes the whole number, and the remainder (1) becomes the numerator: 3 1/3
Conclusion
Therefore, 1 1/3 x 2 1/2 equals 10/3 or 3 1/3.